
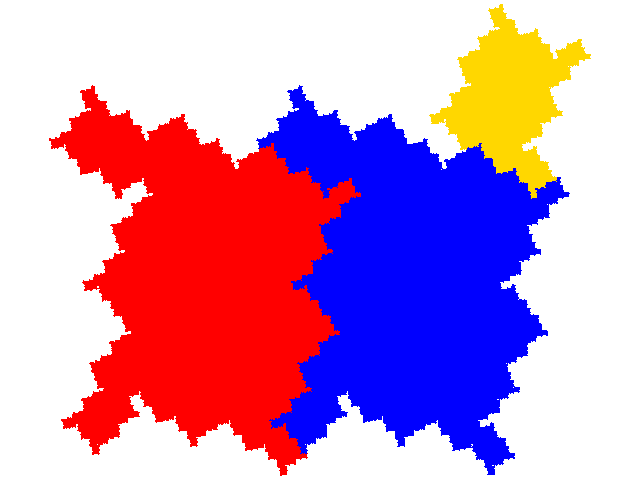
Of the eight order 3 6th cubic tiles, two (the symmetric and windowed
tiles) have the smaller element lying within the larger two elements, two (the
complex teragons) have it lying partially within and
partially outside, and four have it lying outside. Two of these are demi-symmetric tiles, which makes it convenient to refer
to the remaining two as external tiles. The IFS for the first attractor
shown below is { p→-ap; p→-ap + 1;
p→-a3p + 2a2 }. Another IFS, with
an attractor with a different orientation, is {
p→-a3p; p→-ap + 1;
p→-ap + 1 + a2 }. The IFS for the second
attractor is { p→ap; p→ap + 1;
p→a3p + 1 - a-1 - a2
}.


For the first tile there is a tiling with one copy in the unit cell
(signature 0). There are also tilings with other signatures (e.g.
03, 033, 0333). For the tiling with signature 0 the
tiling vectors are 2 + a and a-1. For the
tiling with signature 03 the cell transforms are {
p→p; p→a3p + 1 - a1
- a2 - a3 } and the tiling vectors are 2 +
2a + a-1 and a-1. For the tiling with
signature 033 the cell transforms are { p→p;
p→a3p + 1 - a1 - a2 -
a3; p→a3p + 1 - a2 -
a3 } and the tiling vectors are 2 + 3a +
2a-1 and a-1. For the tiling with
signature 0333 the cell transforms are { p→p;
p→a3p + 1 - a1 - a2 -
a3; p→a3p + 1 - a2 -
a3; p→a3p + 1 - 2a2 -
a3 } and the tiling vectors are 2 + 4a +
2a-1 and a-1. Obviously this series
can be extended for all signatures 03n. Other simple tilings
have signatures 002 (dissecting each unit cell of 0), 0113
(dissecting alternative unit cells along rows, or columns, or diagonals),
0022 (dissecting the larger copy in 03) and 0446
(dissecting the smaller copy in 03).
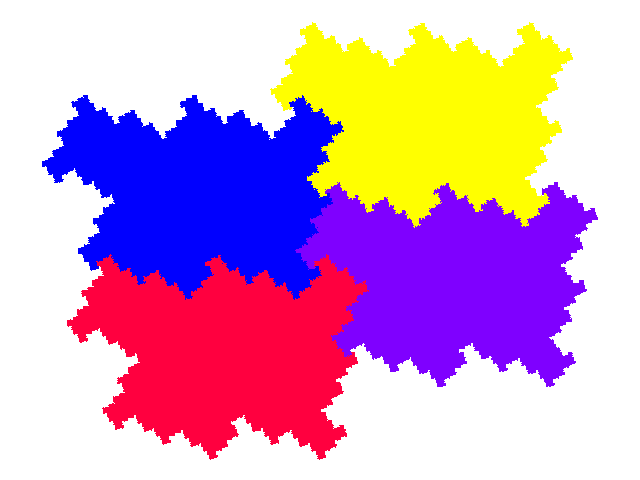
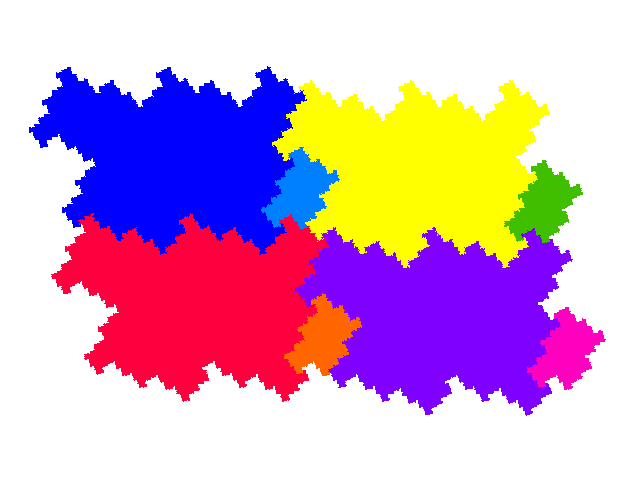
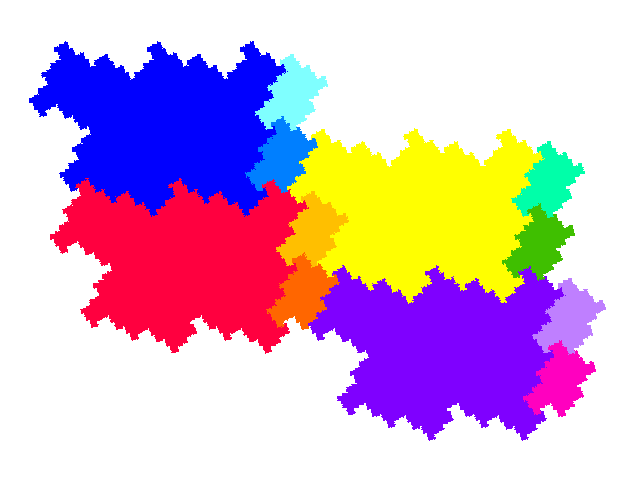

For the second tile there is again a tiling with signature 0. The
tiling vectors are 2 + a and a-1. There
are no obvious "pull apart" tilings, but tilings with signatures like
002 and 0113 can be obtained by dissection.
© 2015, 2016 Stewart R. Hinsley